What I am up to
First a couple of notes: I found some sources that help with math symbols in HTML:
http://barzilai.org/math_sym.htm
http://www.utoronto.ca/ian/books/xhtml1/entity/en_symbol.html
and this is interesting too:
http://www.cs.tut.fi/~jkorpela/math/
I am kind of ashamed that I didn't know this earlier.
Next, while looking for good figures to put in this post (I didn't find them yet), I came across a fun little article which relates elementary knot theory with "impossible diagrams" (Escher type stuff)
http://www.mi.sanu.ac.yu/vismath/cerf/
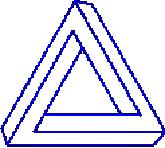 It is a quick, enjoyable read.
It is a quick, enjoyable read.
Now back to the subject at hand: Imagine a knot inside of a torus:

(figure: http://www.rwgrayprojects.com/Lynn/DoubleTorus/dt01.html)
The yellow string of beads is the knot (trefoil). Now suppose that torus (the red solid object) is itself knotted up:
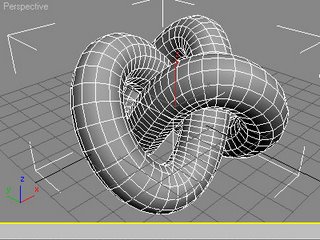
(figure: http://www.imasters.com.br/artigo/1772)
The knot inside the solid torus is called a satellite knot of the knot formed by the solid torus, and the knot formed by the solid torus is called a companion knot of the knot inside the torus. Of course, there is more to the definition than I am telling; for example, the knot inside of the torus (first diagram) must "really" lie in the torus and not in some ball inside of the torus (think of it this way: at the beginning of this process, the knot inside of the torus to be knotted must hit every meridional disk in the beginning torus (the red torus of the first figure). And the example I gave is of a cable knot.
Anyway, at the moment, I am interested in the following kind of object. Consider a knot inside of a companion. Now thicken that knot so that it is a solid torus. Now put another knot inside that. Thicken that knot so that it is a solid torus as well (inside of the two solid tori). Put a knot inside that one, thicken it again, and repeat the process infinitely.
Now take the intersection of this infinite collection of nested tori; kind of like these dolls:
 except that the tori are tied up in more and more complicated fashion.
except that the tori are tied up in more and more complicated fashion.
Now this infinite intersection is an example of what is known as a solenoid.
If we are careful about how we nest these tori; that is, if a longitude of each inner torus just goes once around the torus that contains it, we can get a simple closed curve in the limit.
Arnold Shilepsky came up with criteria that guarantees that we get a simple closed curve in the limit; see the references in his selected publication list. (These types of knots were first studied by a real giant of geometric topology: R. H. Bing), and Shilepsky was one of Bing's students).
These knots are very pathological. They are examples of "wild" knots; we'll say a knot is wild if either it is not the image of any differentiable embedding of the circle into 3-space (as in the sense of ordinary calculus) or if the knot cannot be realized by a finite number of straight line segments placed end to end.
But these knots are not just wild; they are so wild that they fail to "pierce a disk" at any point. Think of it this way: if you think of these knots as pieces of thread, there is no way to run this particular thread through an eye of a needle without running an infinite number of "strands" of this thread though the eye at the same time. That is, if one strand of this type of knot goes through the eye, then an infinite number of strands must go through at the same time as well.
Now the question I am interested in is this: how can one tell if two of these types of wild knots are really different; that is, if space can't be deformed to take one of these wild knots onto the other one?
What symmetries do they have? What about their groups?
http://barzilai.org/math_sym.htm
http://www.utoronto.ca/ian/books/xhtml1/entity/en_symbol.html
and this is interesting too:
http://www.cs.tut.fi/~jkorpela/math/
I am kind of ashamed that I didn't know this earlier.
Next, while looking for good figures to put in this post (I didn't find them yet), I came across a fun little article which relates elementary knot theory with "impossible diagrams" (Escher type stuff)
http://www.mi.sanu.ac.yu/vismath/cerf/
 It is a quick, enjoyable read.
It is a quick, enjoyable read.Now back to the subject at hand: Imagine a knot inside of a torus:

(figure: http://www.rwgrayprojects.com/Lynn/DoubleTorus/dt01.html)
The yellow string of beads is the knot (trefoil). Now suppose that torus (the red solid object) is itself knotted up:

(figure: http://www.imasters.com.br/artigo/1772)
The knot inside the solid torus is called a satellite knot of the knot formed by the solid torus, and the knot formed by the solid torus is called a companion knot of the knot inside the torus. Of course, there is more to the definition than I am telling; for example, the knot inside of the torus (first diagram) must "really" lie in the torus and not in some ball inside of the torus (think of it this way: at the beginning of this process, the knot inside of the torus to be knotted must hit every meridional disk in the beginning torus (the red torus of the first figure). And the example I gave is of a cable knot.
Anyway, at the moment, I am interested in the following kind of object. Consider a knot inside of a companion. Now thicken that knot so that it is a solid torus. Now put another knot inside that. Thicken that knot so that it is a solid torus as well (inside of the two solid tori). Put a knot inside that one, thicken it again, and repeat the process infinitely.
Now take the intersection of this infinite collection of nested tori; kind of like these dolls:
 except that the tori are tied up in more and more complicated fashion.
except that the tori are tied up in more and more complicated fashion.Now this infinite intersection is an example of what is known as a solenoid.
If we are careful about how we nest these tori; that is, if a longitude of each inner torus just goes once around the torus that contains it, we can get a simple closed curve in the limit.
Arnold Shilepsky came up with criteria that guarantees that we get a simple closed curve in the limit; see the references in his selected publication list. (These types of knots were first studied by a real giant of geometric topology: R. H. Bing), and Shilepsky was one of Bing's students).
These knots are very pathological. They are examples of "wild" knots; we'll say a knot is wild if either it is not the image of any differentiable embedding of the circle into 3-space (as in the sense of ordinary calculus) or if the knot cannot be realized by a finite number of straight line segments placed end to end.
But these knots are not just wild; they are so wild that they fail to "pierce a disk" at any point. Think of it this way: if you think of these knots as pieces of thread, there is no way to run this particular thread through an eye of a needle without running an infinite number of "strands" of this thread though the eye at the same time. That is, if one strand of this type of knot goes through the eye, then an infinite number of strands must go through at the same time as well.
Now the question I am interested in is this: how can one tell if two of these types of wild knots are really different; that is, if space can't be deformed to take one of these wild knots onto the other one?
What symmetries do they have? What about their groups?


0 Comments:
Post a Comment
<< Home